MERIT inventor David Harro, PG, first applied it at an apartment building in Tampa, Florida where a sinkhole had swallowed a car. Positive initial results led to Dr Henok Kiflu and Dr Sarah Kruse conducting research at the University of South Florida campus Geopark where several sinkholes are used for research by the Geology department. Research on MERIT continued for several years, which included collaboration from internationally recognized Theoretical Geophysicist Dr M. H. Loke (Getomo Software) and Dr Paul Wilkinson (British Geological Survey).
David Harro is the inventor and patent holder of Multi-Electrical Resistivity Implant Technique (MERIT). He is a professional geologist and drilling contractor with broad range of experience in geophysical methods for karst and environmental applications. Mr Harro worked on the initial theoretical concepts of the Multi-Electrical Resistivity Implant Technique as well as development of its installation process using direct push.
Dr Henok Kiflu graduated with a PhD from the University of South Florida under the supervision of Dr Sarah Kruse. Dr Kiflu completed his doctoral thesis on the Multi-Electrical Resistivity Implant Technique (MERIT) focused on numerical and laboratory studies, field data collection, data processing, data optimization, and theoretical application. His works on MERIT have been published in multiple geophysical journals including the Journal of Applied Geophysics. His research also involves using advanced data science and statistical techniques to study geo-hazard risks. Dr. Kiflu worked as a core R&D member of the GET group where he contributed to the development, field implementation, data management, data analysis and validation of new geophysical techniques such as MERIT-SP and MERIT3D.
Dr. Kiflu also has a strong multi-disciplinary experience in using integrated geophysical, geomechanical and hydrogeological techniques to solve seepage problems in several dam and reservoir sites including Tendaho dam (Logia, Ethiopia) and Kesem dam (Afar, Ethiopia). He has strong experience in geophysics, groundwater modeling, geo-statistics, numerical studies, Geomechanics, laboratory soil analysis, seepage analysis and slope stability analysis.
Dr Sarah Kruse graduated with a PhD from the Massachusetts Institute of Technology in 1989 and is a professor at the University of South Florida. Her research includes Near-Surface Geophysics with applications of ground penetrating radar and electromagnetic, and resistivity methods to environmental studies and volcanological studies as well as numerical simulations of ground penetrating radar response to geologic materials and structures.
Dr M. H. Loke obtained a PhD from the School of Earth Sciences, The University of Birmingham in 1994 under the supervision of Dr. R.D.Barker. The work of Dr Loke and Dr Barker at Birmingham University was among the first to successfully develop solutions to the inverse problem of Electrical Resistivity Tomography (ERT) using computers, and their approach is still widely used.
Dr Loke’s professional papers have over 4000 citations and he is the developer of the RES2DINV and RES3DINV software, and the Director of Geotomo Software. His present research interests are in fast 2D and 3D inversion methods for geo-electrical data, optimization of electrode arrays for 2D and 3D surveys, time-lapse inversion techniques, fast numerical methods and applications of parallel programming techniques in geophysical modelling. He is a member of the editorial board of the Journal of Applied Geophysics and Near Surface Geophysics, and also an invited peer reviewer for the UK Times and QS World University Ranking systems.
Dr Paul Wilkinson received a B.Sc. (Hons.) in Physics from the University of Birmingham in 1993 and a PhD in Theoretical Physics from the University of Nottingham in 1997. He joined the British Geological Survey in 2004 as a theoretical geophysicist. His research interests include geo-electrical tomography, tomographic image optimization, and adaptive experimental design. He is the author of over 140 journal and conference papers
Over the years of research the development of efficient installation procedures, optimization of data collection, improved inversion methods and data validation were fully vetted. The final product of the research resulted in papers published in 13th annual sinkhole conference and two publications in the Journal of Applied Geophysics.
Commercial applications of MERIT have been applied to sinkholes in alignment of roadway to 170 feet by Florida Department of Transportation (FDOT), Geophysical Imaging of geologic conditions to 100 feet below potential location of 5 million gallon water tank for Hillsborough County, Florida , Imaging lateral movement from sinkhole that affected 2 lanes of the Florida Turnpike and threaten a 54 inch pressured reclaim water main for Orlando County Utilities, as well as detail imaging of sinkhole/ downward movement of water near gas pipeline previously surveyed by Electrical Resistivity and Ground Penetrating Radar geophysical surveys for Florida Gas. MERIT has been used by Florida Department of Environmental Protection (FDEP) on former gas stations to show potential contaminated pathways from the upper surface aquifer into the lower main aquifer through sinkholes.
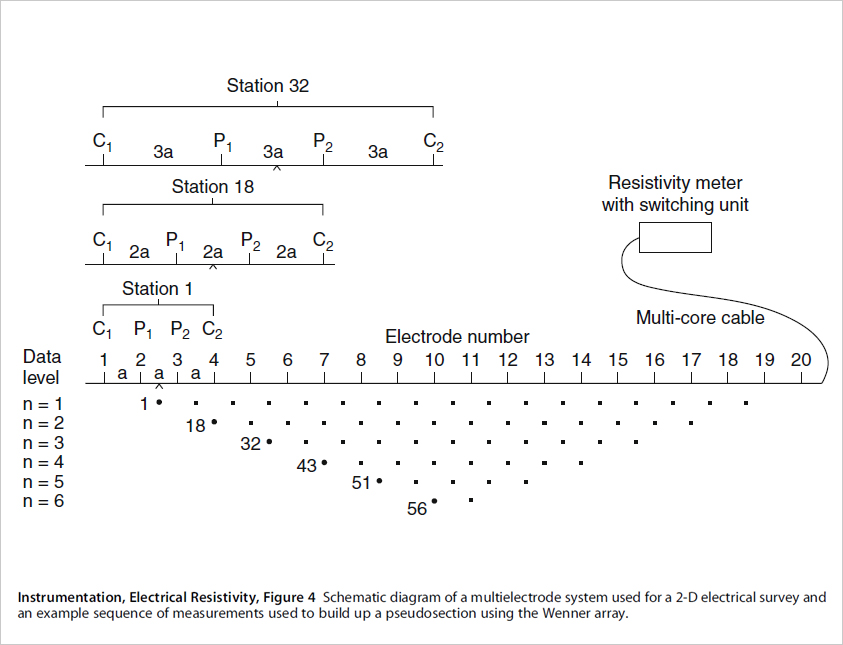
The surface geophysical technique Electrical resistivity is measured by the distribution of electrodes on the surface. This distribution of electrodes creates arrays of transmitters and receivers, typically two electrodes are transmitters and two are receivers. The data collection of the pair of electrodes is performed by geometric progression. Various geometric techniques have been developed using concept of two electrodes as transmitters and two receivers the most common are Wenner, Schlumberger, and Dipole-Dipole. However, all these geometries result in exponential decay of the data into an inverted triangle as shown in the image below.
The data from this arrangement is continually being reduced. As can be seen in the figure, the top line consists of 17 data points out of 20 electrodes positions or 85% of ratio of electrodes to data. Thus the ratio is only 10% of number of electrodes. What this means is at depth the resolution is only 10% of what it is nearer the surface. In addition, the data is not collected along the sides of the inverted triangle, leaving even more data out of the processing. This deficiency of data points greatly affects the data processing into a clear image of the subsurface. The inversion process used to create the image will try to fill in the gaps which can lead to producing artifacts that do not represent the actual subsurface. Existing problems in the inversion or imaging process results in low vertical resolution of the geologic layering or units.
The Multi-Electrical Resistivity Implant Technique uses a distribution of electrodes on the surface and implanted in the subsurface. This creates arrays of transmitters and receivers that can communicate either along each array or between each array using two electrodes as transmitters and two receivers. This combination of surface and implanted arrays provides the essential components of spacing and geometric patterns of data collection that is significantly higher than what can be achieved using only a surface array.
The sensitivity analysis performed on a surface Dipole-Dipole array shown left in the figure above is a normal electrode resistivity survey consisting of 250 data points. The sensitivity scale shows the highest location of quality data collected. As can be seen in the Dipole-Dipole array the majority of the quality data is near the surface above 5 meter depth.
The sensitivity analysis performed on a surface Dipole-Dipole array shown left in the figure above is a normal electrode resistivity survey consisting of 250 data points. The sensitivity scale shows the highest location of quality data collected. As can be seen in the Dipole-Dipole array the majority of the quality data is near the surface above 5 meter depth.
Another example is how well MERIT methods can locate a target. Shown here is a forward model of targets with a background electrical resistivity value of 500 Ohm-meters, Targets of similar size but different electrical resistivity values 500 and 1000 Ohm-meters were placed at different depths and were simulated (Upper Image). The image in the middle is the result of using standard surface electrical resistivity techniques, while the lower image is MERIT results. It can be clearly seen that all the targets, their depths, and relative size can be seen using MERIT.